Discovery and design of novel materials in silico: current state of the field

By Prof. Oleg Yazyev, EPFL
First-principles computer simulations are playing a crucial role in condensed matter physics for already several decades. The application domain of such computations is currently undergoing dramatic extension enabled by automated computational workflows, open science culture, machine learning and other recent developments. In this Scientific Perspective, I would like to express my view on computations as an instrument for the discovery and design of new materials. I will give a few examples of interesting materials discovered in silico (i.e. on computer) in my group and later verified experimentally. I will also present open-science tools developed by NCCR Marvel “Materials’ revolution: Computational Design and Discovery of Novel Materials”.
Materials discovery by high-throughput screening
The capabilities of first-principles computer simulations have been undergoing fast-paced extension due to the progress along several directions. First, computer hardware has been following Moore’s Law. High-performance computing (HPC) resources are becoming increasingly standardized and are now readily available at user facilities such as the Swiss National Supercomputing Center (CSCS). Second, first-principles methods are becoming more elaborate and accurate thanks to the efforts of a large community of researchers. In this diverse ecosystem of methodologies, density functional theory (DFT) has become the workhorse technique in condensed matter physics. Third, a large number of computer codes, most of which are open-source, implementing these methods is currently available, again as a result of years of hard work of numerous academic research groups. These codes are getting more reliable and easier to use. Knowing how to perform DFT calculations has become a basic skill for condensed matter physics researchers well outside the specialized computational groups. While DFT is able to predict a large number of ground-state (i.e. structural, vibrational, magnetic, etc.) properties with reasonable accuracy at very modest computational cost, it’s certainly not a good instrument for studying excited-state properties (band gaps, excitons, etc.) and correlated systems. In such situations, one has to resort to higher-level methods such as the GW approximation, dynamical mean-field theory, quantum Monte Carlo, etc. These tools are computationally more (usually much more or prohibitively more) expensive and require a deep understanding of their foundations, but nevertheless are mature enough to be applied to a broad range of problems in condensed matter physics.
The progress in the availability and the predictive power of first-principles calculations has led to a straightforward idea of replacing actual experiments with simulations. Calculations can be performed much faster and at much lower cost. Furthermore, calculations do not suffer from possible problems that experimental researchers have to deal with, e.g. the inability to grow crystals of sufficiently good quality. Of course, simulations (read: experiments in silico) cannot be considered a full equivalent of real experiments, but nevertheless numerical work can help identifying the most promising materials for performing experiments. We call such materials candidate materials. This first step carried out in silico can accelerate tremendously materials discovery that aims at finding compounds with novel or superior properties – magnetic, superconducting, topological, optical, etc. – name your favorite. A candidate material becomes real discovery only once its properties predicted in silico are confirmed by experiments.
Eventually, one can perform first-principles calculations on very large collections of materials in a highly automated way. In this case we are speaking about high-throughput screening. The natural starting point for materials discovery in condensed matter physics would be the databases of crystal structures of inorganic materials obtained from x-ray diffraction experiments. The two widely known examples are Inorganic Crystal Structure Database (ICSD) [1] and Crystallography Open Database (COD) [2]. A less known source of data is the Pauling File database [3,4]. In addition to crystal structures, this database contains phase diagrams and a wide range of physical properties (e.g. temperatures of various phase transitions) extracted from publications – a highly precious knowledge in the context of materials discovery. Combining data from these sources results in several hundreds of thousands of crystal structure entries – not really an impressive number by the modern big data standards. Depending on the nature of the problem, the data set can be further reduced by a pre-screening filter, e.g. to eliminate crystal structures with fractional occupations that cannot be easily handled in first-principles calculations, or to restrict the scope to desired ranges of chemical composition, space groups, etc. The data set is now ready for performing automated first-principles calculations, which is the time-consuming part of work, and the results are in turn organized for further processing (see Fig. 1).

Different high-throughput screening workflows and databases of results have been built by the community for different classes of materials. Below, I will focus only on materials discovery efforts in the field of topological materials, in which my group is active. This field has been rapidly expanding during the past 15 years resulting in the discovery of numerous topological electronic phases (topological insulators, Dirac and Weyl semimetals, nodal-line semimetals, etc.) and materials hosting them. Importantly, theory and computations have been playing the leading role in this field. This is mostly for the following two reasons. Firstly, standard density functional theory is able to provide rather accurate description of non-interacting topological phases. Secondly, the results of such calculations are indispensable in guiding follow-up experiments, e.g. providing information about the dispersion of surface states expected to be observed in ARPES measurements. The vast majority of discovered topological materials have first been identified by means of first-principles calculations. For example, the first three-dimensional Dirac semimetals Na3Bi [5] and Cd3As2 [6] have been discovered following the respective theoretical predictions of Refs. [7] and [8]. Similarly, experimental works on the first Weyl semimetal TaAs [10,11] were preceded by the theoretical prediction [12].
High-throughput computational search of novel topological materials performed by different groups and consortia has resulted in several online databases of results such as Topological Materials Database from Princeton and Basque Country [13], Materiae from Beijing [14] and the TopoMat database [15] created in my group by Dr. Gabriel Autès. I would like to apologize for the incompleteness of this list; several other databases of candidate topological materials can easily be found on internet. Importantly, these databases containing big volumes of results (topological indices, band structures, etc.) can be accessed for free and without any registration. Everyone is welcome to use the data without asking for permission (even though we would be glad to hear if our data has helped someone to discover an interesting material). This is the essence of open science, which is rapidly becoming a norm in the academic community. Our database is hosted on the Materials Cloud portal [16], one of the infrastructure pillars of NCCR Marvel. I would like to invite all members of MaNEP to explore the content of Materials Cloud (//www.materialscloud.org) for other databases (2D materials, metal organic frameworks, covalent organic frameworks, etc.), online tools, archived data and educational materials. New content is being added on a daily basis.
While I believe many topological materials will be discovered with the help of our database by other groups, we had a privilege to harvest ourselves some low-hanging fruits. One example is the discovery of the topological insulator phase in bismuth iodide -Bi4I4. This material was first described decades ago [17] without attracting much interest afterwards. Our prediction of the topological insulator phase in -Bi4I4 was motivating enough to easily establish collaborations with experimental groups. In particular, the samples of -Bi4I4 were provided by Dr. Anna Isaeva (TU Dresden), while the ARPES measurements performed by Dr. Luca Moreschini at the ALS synchrotron facility in Berkeley provided an unambiguous confirmation of the predicted topological insulator phase (Fig. 2). This material appeared to be very different from other topological insulators known by that time (bismuth chalcogenides and their ternary derivatives), in particular showing highly anisotropic Dirac fermion surface states and low concentration of intrinsic charge carriers. Our prediction, synthesis and characterization of the samples as well as the ARPES measurements were published in one single paper [18], which demonstrates what I prefer calling the full cycle of materials discovery. This also gave us, theorists, an exciting experience of coordinating experimental activities carried out in different labs across the globe.

Our another contribution to the field of topological materials was the discovery of two Weyl semimetals MoP2 and WP2. Once again, after being first described in 1963 [19], these materials remained in the shadow until five years ago when they got spotted by Gabriel Autés and Alexey Soluyanov when scrolling through the list of candidate materials in the TopoMat database. The unique feature of MoP2 and WP2 is the unusually large distance in momentum space between the Weyl points of opposite chirality (for this reason we called them robust Weyl semimetals), and hence many responses resulting from the Weyl semimetal phase were expected to manifest stronger than in known Weyl semimetals. Motivated by our publication [20], many experimental groups have soon published their studies of these new Weyl semimetals. Early publications revealed a combination of extraordinary properties of WP2 [21], including exceptionally large non-saturating magnetoresistance (2×108 %, albeit at 63 T and 0.5 K), very high low-temperature conductivity and residual resistivity ratio (RRR ~ 25000), very long mean free path as well as the observation of hydrodynamic transport at low temperatures [22]. It remains to be understood how all these properties are related to the Weyl semimetal phase, but nevertheless the presence of the Weyl node degeneracies was confirmed by the ARPES measurements [23], while the non-saturating transverse magnetoresistance was ascribed to the ordinary charge-carrier compensation effect [24].
Outlook for materials design
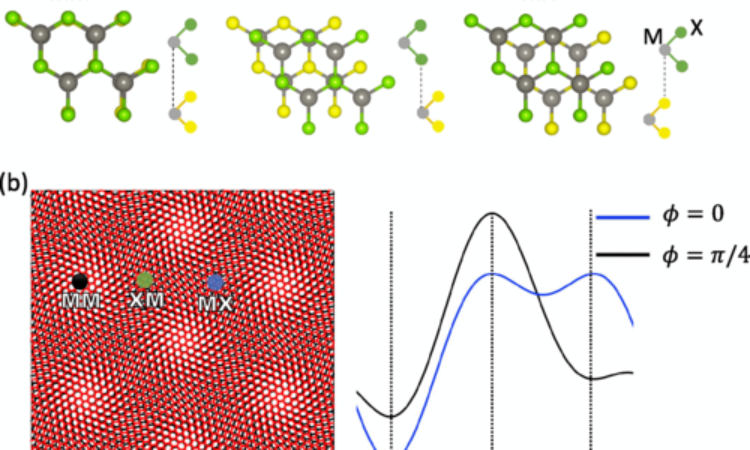
While many materials with extraordinary properties remain to be discovered by performing high-throughput screening of already known crystal structures, but the question of designing materials that have never been synthesized previously remains largely open. Numerous computational methods allowing to predict novel crystal structures already exist, and are especially useful in elucidating high-pressure phases of materials. However, there is no universal tool that would be able to tell which growth technique and conditions have to be used for growing crystals of a predicted material. Perhaps, machine learning techniques can help addressing this problem in the nearest future. But for now, true design of crystalline materials is possible only within certain classes of materials. One such example is covalent organic frameworks (COFs) which rely on practically unlimited assortment of organic precursor molecules and several trusted chemical reactions for assembling the designed crystal structure. Another example are the heterostructures of 2D materials, in which a rather broad library of individual 2D monolayers is combined with the freedom of arranging them in arbitrary order by various transfer techniques, i.e. literally by hand. Furthermore, the twist degree of freedom, which is another consequence of reduced dimensionality of 2D materials, turned out to be a powerful instrument for tuning the properties of such heterostructures after the discovery of superconductivity in magic-angle twisted bilayer graphene [25]. The moiré superlattice potential resulting from the relative twist of layers is a straightforward way of designing crystalline lattices with large periodicity. As a final remark, it is worth stressing that high-throughput computational screening is currently playing an important role in guiding the design of moiré superlattice systems.
Acknowledgment. The work carried out in my group that I mentioned above would never be completed without contributions of several members of my group, but I would like to specially thank my postdocs Gabriel Autès and Quansheng Wu. Close collaboration with Alexey Soluyanov, who will remain in our memories, was instrumental for our projects on topological materials. I would like to thank also Nicola Marzari and the members of his team. I am also grateful to our experimental colleagues, in particular to Anna Isaeva in Dresden, Luca Moreschini in Berkeley, Ming Shi and the members of his team (PSI) as well as to numerous colleagues at EPFL (Marco Grioni, Alberto Crepaldi, Jens Johannsen, László Forró, Andrea Pisoni, Arnaud Magrez). The financials support is from NCCR Marvel, ERC and Swiss NSF.
References:
[2] //www.crystallography.net/cod/search.html
[4] //mpds.io
[5] Liu, Z. K. et al. Discovery of a Three-Dimensional Topological Dirac Semimetal Na3Bi. Science 343, 864-867 (2014). [//doi.org/10.1126/science.1245085]
[6] Liu, Z. K. et al. A stable three-dimensional topological Dirac semimetal Cd3As2. Nature Materials 13, 677-681 (2014). [//doi.org/10.1038/nmat3990]
[7] Wang, Z. et al. Dirac semimetal and topological phase transitions in A3Bi (A=Na, K, Rb). Phys. Rev. B 85, 195320 (2012). [//doi.org/10.1103/PhysRevB.85.195320]
[8] Wang, Z., Weng, H., Wu, Q., Dai, X. & Fang, Z. Three-dimensional Dirac semimetal and quantum transport in Cd3As2. Phys. Rev. B 88, 125427 (2013). [//doi.org/10.1103/PhysRevB.88.125427]
[10] Xu, S.-Y. et al. Discovery of a Weyl fermion semimetal and topological Fermi arcs. Science 349, 613-617 (2015). [//doi.org/10.1126/science.aaa9297]
[11] Lv, B. Q. et al. Experimental Discovery of Weyl Semimetal TaAs. Phys. Rev. X 5, 031013 (2015). [//doi.org/10.1103/PhysRevX.5.031013]
[12] Weng, H., Fang, C., Fang, Z., Bernevig, B. A. & Dai, X. Weyl Semimetal Phase in Noncentrosymmetric Transition-Metal Monophosphides. Phys. Rev. X 5, 011029 (2015). [//doi.org/10.1103/PhysRevX.5.011029]
[13] //www.topologicalquantumchemistry.com
[15] Autès, G., Wu, Q., Mounet, N. & Yazyev, O. V. TopoMat: a Database of High-Throughput First-Principles Calculations of Topological Materials. [//www.materialscloud.org/discover/topomat]
[16] Talirz, L. et al. Materials Cloud, a platform for open computational science. Scientific Data 7, 299 (2020). [//doi.org/10.1038/s41597-020-00637-5]
[17] von Schnering, H. G., von Benda, H. & Kalveram, C. Wismutmonojodid BiJ, eine Verbindung mit Bi(O) und Bi(II). Z. Anorg. Allgem. Chem. 438, 37-52 (1978). [//doi.org/10.1002/zaac.19784380104]
[18] Autès, G. et al. A novel quasi-one-dimensional topological insulator in bismuth iodide -Bi4I4. Nature Materials 15, 154-158 (2016). [//doi.org/10.1038/nmat4488]
[19] Rundqvist, R. & Lundström, T. X-Ray Studies of Molybdenum and Tungsten Phosphides. Acta Chem. Scand. 17, 37 (1963). [//doi.org/10.3891/acta.chem.scand.17-0037]
[20] Autès, G., Gresch, D., Troyer, M., Soluyanov, A. A. & Yazyev, O. V. Robust Type-II Weyl Semimetal Phase in Transition Metal Diphosphides XP2 (X = Mo, W). Phys. Rev. Lett. 117, 066402 (2016). [//doi.org/10.1103/PhysRevLett.117.066402]
[21] Kumar, N. et al. Extremely high magnetoresistance and conductivity in the type-II Weyl semimetals WP2 and MoP2. Nature Communications 8, 1642 (2017). [//doi.org/10.1038/s41467-017-01758-z]
[22] Gooth, J. et al. Thermal and electrical signatures of a hydrodynamic electron fluid in tungsten diphosphide. Nature Communications 9, 4093 (2018). [//doi.org/10.1038/s41467-018-06688-y]
[23] Yao, M. Y. et al. Observation of Weyl Nodes in Robust Type-II Weyl Semimetal WP2. Phys. Rev. Lett. 122, 176402 (2019). [//doi.org/10.1103/PhysRevLett.122.176402]
[24] Zhang, S., Wu, Q., Liu, Y. & Yazyev, O. V. Magnetoresistance from Fermi surface topology. Phys. Rev. B 99, 035142 (2019). [//doi.org/10.1103/PhysRevB.99.035142]
[25] Cao, Y. et al. Unconventional superconductivity in magic-angle graphene superlattices. Nature 556, 43 (2018). [//doi.org/10.1038/nature26160]